- 1.5 Multiplying Whole Numbers Page 31 1.6 Dividing Whole Numbers (Part I) Page 42 1.7 Dividing Whole Numbers (Part II) Page 48 1.8 Exponents, square roots, and the order of operations Page 56 Chapter 2 2.1 Divisibility tests, factors, and primes Page 65 2.2 Introduction to fractions Page 74 2.3 Reducing fractions Page 84 2.4 Multiplying.
- Various contexts and models are introduced to help students make sense of when multiplication is appropriate.In Problem 2.1 students develop an understanding of multiplication with simple fractions. Problems 2.2 and 2.3 focus on multiplication with fraction, mixed number, and whole-number combinations.
- Multiply a fraction and a whole number! Solve problems involving the multiplication of a fraction and a whole number. pattern blocks Understanding Multiplication The product of 4 and 2 is 8, because 4 × 2 = 8. The equation 4 × 2 = 8 means that 4 groups of 2 make 8. You can also think of 4 × 2 as the repeated addition 2 + 2 + 2 + 2.
Year 2 - Model Text - Poetry - A Concrete Poem Model poems from a set of whole school model. Mrs mac's classroom website.
Standards in this domain:
Use equivalent fractions as a strategy to add and subtract fractions.
CCSS.Math.Content.5.NF.A.1
Add and subtract fractions with unlike denominators (including mixed numbers) by replacing given fractions with equivalent fractions in such a way as to produce an equivalent sum or difference of fractions with like denominators. For example, 2/3 + 5/4 = 8/12 + 15/12 = 23/12. (In general, a/b + c/d = (ad + bc)/bd.)
Add and subtract fractions with unlike denominators (including mixed numbers) by replacing given fractions with equivalent fractions in such a way as to produce an equivalent sum or difference of fractions with like denominators. For example, 2/3 + 5/4 = 8/12 + 15/12 = 23/12. (In general, a/b + c/d = (ad + bc)/bd.)
CCSS.Math.Content.5.NF.A.2
Solve word problems involving addition and subtraction of fractions referring to the same whole, including cases of unlike denominators, e.g., by using visual fraction models or equations to represent the problem. Use benchmark fractions and number sense of fractions to estimate mentally and assess the reasonableness of answers. For example, recognize an incorrect result 2/5 + 1/2 = 3/7, by observing that 3/7 < 1/2.
Solve word problems involving addition and subtraction of fractions referring to the same whole, including cases of unlike denominators, e.g., by using visual fraction models or equations to represent the problem. Use benchmark fractions and number sense of fractions to estimate mentally and assess the reasonableness of answers. For example, recognize an incorrect result 2/5 + 1/2 = 3/7, by observing that 3/7 < 1/2.
Apply and extend previous understandings of multiplication and division.
CCSS.Math.Content.5.NF.B.3
Interpret a fraction as division of the numerator by the denominator (a/b = a ÷ b). Solve word problems involving division of whole numbers leading to answers in the form of fractions or mixed numbers, e.g., by using visual fraction models or equations to represent the problem. For example, interpret 3/4 as the result of dividing 3 by 4, noting that 3/4 multiplied by 4 equals 3, and that when 3 wholes are shared equally among 4 people each person has a share of size 3/4. If 9 people want to share a 50-pound sack of rice equally by weight, how many pounds of rice should each person get? Between what two whole numbers does your answer lie?
Interpret a fraction as division of the numerator by the denominator (a/b = a ÷ b). Solve word problems involving division of whole numbers leading to answers in the form of fractions or mixed numbers, e.g., by using visual fraction models or equations to represent the problem. For example, interpret 3/4 as the result of dividing 3 by 4, noting that 3/4 multiplied by 4 equals 3, and that when 3 wholes are shared equally among 4 people each person has a share of size 3/4. If 9 people want to share a 50-pound sack of rice equally by weight, how many pounds of rice should each person get? Between what two whole numbers does your answer lie?
CCSS.Math.Content.5.NF.B.4
Apply and extend previous understandings of multiplication to multiply a fraction or whole number by a fraction.
Apply and extend previous understandings of multiplication to multiply a fraction or whole number by a fraction.
CCSS.Math.Content.5.NF.B.4.a
Interpret the product (a/b) × q as a parts of a partition of q into b equal parts; equivalently, as the result of a sequence of operations a × q ÷ b. For example, use a visual fraction model to show (2/3) × 4 = 8/3, and create a story context for this equation. Do the same with (2/3) × (4/5) = 8/15. (In general, (a/b) × (c/d) = (ac)/(bd).
Interpret the product (a/b) × q as a parts of a partition of q into b equal parts; equivalently, as the result of a sequence of operations a × q ÷ b. For example, use a visual fraction model to show (2/3) × 4 = 8/3, and create a story context for this equation. Do the same with (2/3) × (4/5) = 8/15. (In general, (a/b) × (c/d) = (ac)/(bd).
CCSS.Math.Content.5.NF.B.4.b
Find the area of a rectangle with fractional side lengths by tiling it with unit squares of the appropriate unit fraction side lengths, and show that the area is the same as would be found by multiplying the side lengths. Multiply fractional side lengths to find areas of rectangles, and represent fraction products as rectangular areas.
Find the area of a rectangle with fractional side lengths by tiling it with unit squares of the appropriate unit fraction side lengths, and show that the area is the same as would be found by multiplying the side lengths. Multiply fractional side lengths to find areas of rectangles, and represent fraction products as rectangular areas.
CCSS.Math.Content.5.NF.B.5
Interpret multiplication as scaling (resizing), by:
Interpret multiplication as scaling (resizing), by:
CCSS.Math.Content.5.NF.B.5.a
Comparing the size of a product to the size of one factor on the basis of the size of the other factor, without performing the indicated multiplication.
Comparing the size of a product to the size of one factor on the basis of the size of the other factor, without performing the indicated multiplication.
2.2 Multiply A Fraction By A Whole Numbermr. Mac's Page Printable
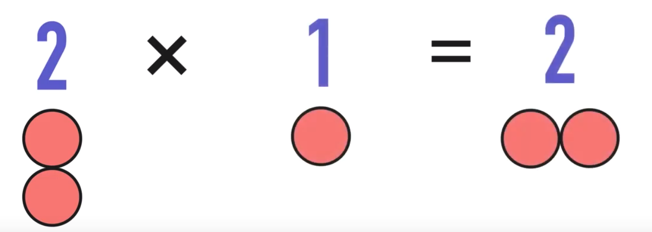
Standards in this domain:
Use equivalent fractions as a strategy to add and subtract fractions.
CCSS.Math.Content.5.NF.A.1
Add and subtract fractions with unlike denominators (including mixed numbers) by replacing given fractions with equivalent fractions in such a way as to produce an equivalent sum or difference of fractions with like denominators. For example, 2/3 + 5/4 = 8/12 + 15/12 = 23/12. (In general, a/b + c/d = (ad + bc)/bd.)
Add and subtract fractions with unlike denominators (including mixed numbers) by replacing given fractions with equivalent fractions in such a way as to produce an equivalent sum or difference of fractions with like denominators. For example, 2/3 + 5/4 = 8/12 + 15/12 = 23/12. (In general, a/b + c/d = (ad + bc)/bd.)
CCSS.Math.Content.5.NF.A.2
Solve word problems involving addition and subtraction of fractions referring to the same whole, including cases of unlike denominators, e.g., by using visual fraction models or equations to represent the problem. Use benchmark fractions and number sense of fractions to estimate mentally and assess the reasonableness of answers. For example, recognize an incorrect result 2/5 + 1/2 = 3/7, by observing that 3/7 < 1/2.
Solve word problems involving addition and subtraction of fractions referring to the same whole, including cases of unlike denominators, e.g., by using visual fraction models or equations to represent the problem. Use benchmark fractions and number sense of fractions to estimate mentally and assess the reasonableness of answers. For example, recognize an incorrect result 2/5 + 1/2 = 3/7, by observing that 3/7 < 1/2.
Apply and extend previous understandings of multiplication and division.
CCSS.Math.Content.5.NF.B.3
Interpret a fraction as division of the numerator by the denominator (a/b = a ÷ b). Solve word problems involving division of whole numbers leading to answers in the form of fractions or mixed numbers, e.g., by using visual fraction models or equations to represent the problem. For example, interpret 3/4 as the result of dividing 3 by 4, noting that 3/4 multiplied by 4 equals 3, and that when 3 wholes are shared equally among 4 people each person has a share of size 3/4. If 9 people want to share a 50-pound sack of rice equally by weight, how many pounds of rice should each person get? Between what two whole numbers does your answer lie?
Interpret a fraction as division of the numerator by the denominator (a/b = a ÷ b). Solve word problems involving division of whole numbers leading to answers in the form of fractions or mixed numbers, e.g., by using visual fraction models or equations to represent the problem. For example, interpret 3/4 as the result of dividing 3 by 4, noting that 3/4 multiplied by 4 equals 3, and that when 3 wholes are shared equally among 4 people each person has a share of size 3/4. If 9 people want to share a 50-pound sack of rice equally by weight, how many pounds of rice should each person get? Between what two whole numbers does your answer lie?
CCSS.Math.Content.5.NF.B.4
Apply and extend previous understandings of multiplication to multiply a fraction or whole number by a fraction.
Apply and extend previous understandings of multiplication to multiply a fraction or whole number by a fraction.
CCSS.Math.Content.5.NF.B.4.a
Interpret the product (a/b) × q as a parts of a partition of q into b equal parts; equivalently, as the result of a sequence of operations a × q ÷ b. For example, use a visual fraction model to show (2/3) × 4 = 8/3, and create a story context for this equation. Do the same with (2/3) × (4/5) = 8/15. (In general, (a/b) × (c/d) = (ac)/(bd).
Interpret the product (a/b) × q as a parts of a partition of q into b equal parts; equivalently, as the result of a sequence of operations a × q ÷ b. For example, use a visual fraction model to show (2/3) × 4 = 8/3, and create a story context for this equation. Do the same with (2/3) × (4/5) = 8/15. (In general, (a/b) × (c/d) = (ac)/(bd).
CCSS.Math.Content.5.NF.B.4.b
Find the area of a rectangle with fractional side lengths by tiling it with unit squares of the appropriate unit fraction side lengths, and show that the area is the same as would be found by multiplying the side lengths. Multiply fractional side lengths to find areas of rectangles, and represent fraction products as rectangular areas.
Find the area of a rectangle with fractional side lengths by tiling it with unit squares of the appropriate unit fraction side lengths, and show that the area is the same as would be found by multiplying the side lengths. Multiply fractional side lengths to find areas of rectangles, and represent fraction products as rectangular areas.
CCSS.Math.Content.5.NF.B.5
Interpret multiplication as scaling (resizing), by:
Interpret multiplication as scaling (resizing), by:
CCSS.Math.Content.5.NF.B.5.a
Comparing the size of a product to the size of one factor on the basis of the size of the other factor, without performing the indicated multiplication.
Comparing the size of a product to the size of one factor on the basis of the size of the other factor, without performing the indicated multiplication.
2.2 Multiply A Fraction By A Whole Numbermr. Mac's Page Printable
CCSS.Math.Content.5.NF.B.5.b
Explaining why multiplying a given number by a fraction greater than 1 results in a product greater than the given number (recognizing multiplication by whole numbers greater than 1 as a familiar case); explaining why multiplying a given number by a fraction less than 1 results in a product smaller than the given number; and relating the principle of fraction equivalence a/b = (n × a)/(n × b) to the effect of multiplying a/b by 1.
Explaining why multiplying a given number by a fraction greater than 1 results in a product greater than the given number (recognizing multiplication by whole numbers greater than 1 as a familiar case); explaining why multiplying a given number by a fraction less than 1 results in a product smaller than the given number; and relating the principle of fraction equivalence a/b = (n × a)/(n × b) to the effect of multiplying a/b by 1.
CCSS.Math.Content.5.NF.B.6
Solve real world problems involving multiplication of fractions and mixed numbers, e.g., by using visual fraction models or equations to represent the problem.
Solve real world problems involving multiplication of fractions and mixed numbers, e.g., by using visual fraction models or equations to represent the problem.
CCSS.Math.Content.5.NF.B.7
Apply and extend previous understandings of division to divide unit fractions by whole numbers and whole numbers by unit fractions.1
Apply and extend previous understandings of division to divide unit fractions by whole numbers and whole numbers by unit fractions.1
CCSS.Math.Content.5.NF.B.7.a
Interpret division of a unit fraction by a non-zero whole number, and compute such quotients. For example, create a story context for (1/3) ÷ 4, and use a visual fraction model to show the quotient. Use the relationship between multiplication and division to explain that (1/3) ÷ 4 = 1/12 because (1/12) × 4 = 1/3.
Interpret division of a unit fraction by a non-zero whole number, and compute such quotients. For example, create a story context for (1/3) ÷ 4, and use a visual fraction model to show the quotient. Use the relationship between multiplication and division to explain that (1/3) ÷ 4 = 1/12 because (1/12) × 4 = 1/3.
2.2 Multiply A Fraction By A Whole Numbermr. Mac's Page Sheet
CCSS.Math.Content.5.NF.B.7.b
Interpret division of a whole number by a unit fraction, and compute such quotients. For example, create a story context for 4 ÷ (1/5), and use a visual fraction model to show the quotient. Use the relationship between multiplication and division to explain that 4 ÷ (1/5) = 20 because 20 × (1/5) = 4.
Interpret division of a whole number by a unit fraction, and compute such quotients. For example, create a story context for 4 ÷ (1/5), and use a visual fraction model to show the quotient. Use the relationship between multiplication and division to explain that 4 ÷ (1/5) = 20 because 20 × (1/5) = 4.
CCSS.Math.Content.5.NF.B.7.c
Solve real world problems involving division of unit fractions by non-zero whole numbers and division of whole numbers by unit fractions, e.g., by using visual fraction models and equations to represent the problem. For example, how much chocolate will each person get if 3 people share 1/2 lb of chocolate equally? How many 1/3-cup servings are in 2 cups of raisins?
Solve real world problems involving division of unit fractions by non-zero whole numbers and division of whole numbers by unit fractions, e.g., by using visual fraction models and equations to represent the problem. For example, how much chocolate will each person get if 3 people share 1/2 lb of chocolate equally? How many 1/3-cup servings are in 2 cups of raisins?
2.2 Multiply A Fraction By A Whole Numbermr. Mac's Page Numbers
1Students able to multiply fractions in general can develop strategies to divide fractions in general, by reasoning about the relationship between multiplication and division. Home for the holidays trailer. But division of a fraction by a fraction is not a requirement at this grade.
The main objective of the crowdfunding video is for you to get the investment. So, if you don't ask for the investment, you simply just won't get it. When asking investors to invest in your idea, you need to think about how you ask, what would sell you to invest? Ensure that the ask is sincere, carefully worded and clear. The art of the crowdfunding video production. With advent of crowdfunding, film and video makers now have the option to seek funding directly from their audiences. This gives them the freedom to find funding in a time-efficient manner and still maintain their creative integrity — a first in the media industry bound to produce exciting results. A Little Bit of Background. Crowdfunding expert Chris Hawker says a video is typically the first thing a potential supporter views on a website. 'The video is absolutely the most important piece of the campaign beyond the product and the marketing strategy,' says Hawker, who has used crowdfunding to raise money for his own inventions and served as a crowdfunding.
